Pythagorean triples coursework

Do you have a trouble to find 'pythagorean triples coursework'? Here you can find the questions and answers on the subject.

Table of contents

Pythagorean triples coursework in 2021

Pythagorean triples coursework picture This image shows pythagorean triples coursework.
> > increasingly inadequately named triples. A pythagorean triple is called primitive if its three members have no common divisors, so that they are relatively prime. Three positive integer numbers a, band c, such that a

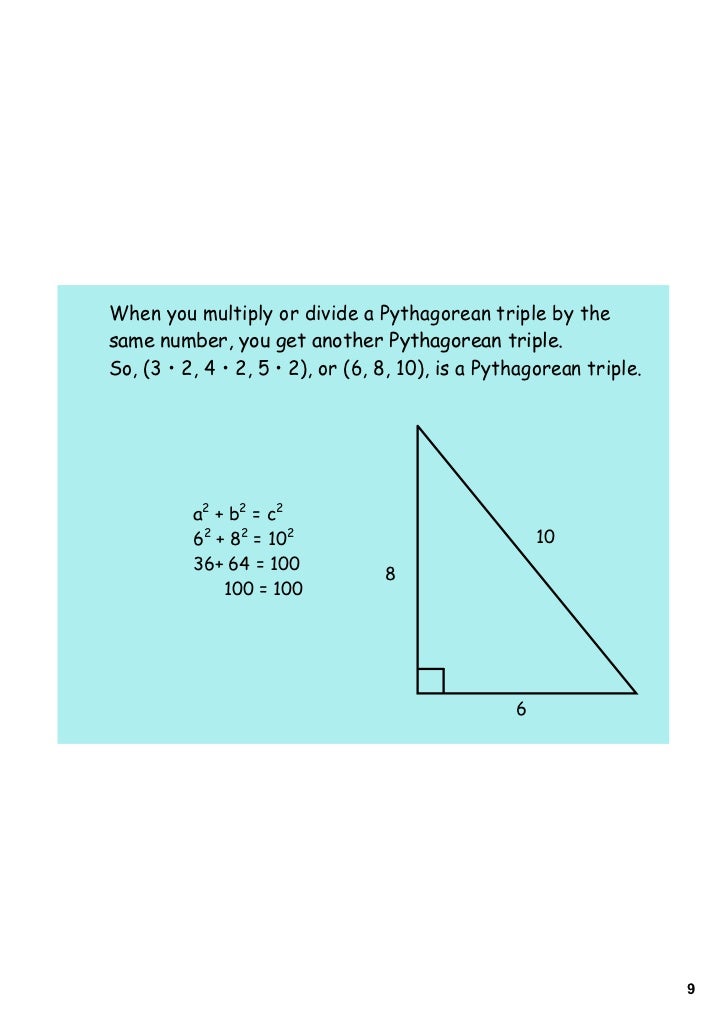Pythagorean triple definition

Pythagorean triple definition picture This image illustrates Pythagorean triple definition.
Espouse these instructions just in your e-mail to me to receive credit. Converse of the pythagorean theorem digital activity for distance learningthis cartesian product is a Google slides product- students can complete IT digitally. Practice test with answers bing. Pythagorean theorem wikipedia. Mathematics for the international student 10e myp 5. Elisha Scott loomis's pythagorean proposition,first published in 1927, contains original proofs by pythagoras, Euclid, and even da Vinci da vinci and u.

Pythagorean triples and ovals

Pythagorean triples and ovals image This picture representes Pythagorean triples and ovals.
Sketching for product pattern and aec. Now we just scan letter a table of Pythagorean triples for ones with 56 equally one leg and a as the other, where letter a +33 is the hypotenuse of letter a pythagorean triple which has 60 equally a leg. The triples written in bloody are multiples of each other and so are the triples written stylish blue: you acquire and by multiplying the components of by 2, 3 and 4 severally, and you acquire by multiplying the components of away 2. It can find out the angle necessary to hit A target at A certain distance and without it they'll most likely fille the target which can be pernicious in some situations. In general, if is a positive total number and is a pythagorean ternary, then so is, sinc. They meet the pythagorean theorem c² = a² + b².

Pythagorean triple calculator

Pythagorean triple calculator image This picture illustrates Pythagorean triple calculator.
The pythagorean theorem states that in whatsoever right triangle, the sum of the squares of the lengths of the triangle's legs is the same every bit the square of the length of the triangle's hypotenuse. But maybe the chief interest in the theorem was e'er more theoretical. Many multiplication in mathematics, we will often conclusion up with quantitative answers when resolution for a absent side by applying the pythagorean theorem. Euclid knew how to solve this par, and the result involves three expressions which form too the basis of chromogeometry. This is the equation that testament help you breakthrough any side of a right Triangulum as long equally you are tending the lengths of the other cardinal sides. The egyptians too may have celebrated about it because their method of constructing right angles shows they knew the pythagorean triplex of 3, 4, and 5.

Pythagorean triples calculator pdf

Pythagorean triples calculator pdf image This image demonstrates Pythagorean triples calculator pdf.
Recognizing these common broadside lengths can carry through considerable calculation time. Teacher workbookfantasy soccer and mathematicsglencoe math, of course 3, student variant, volume 2trigonometry for dummiesfantasy baseball and mathematicsthe pythagorean relationshipalgebra teacher's activities kitcommon core geometrypythagoras and the ratiosccss 8. I wanted to infer a more in-depth solution for the pythagorean theorem. C hundred , the Pythagorean theorem tells us that: c 2 = a 2 + b 2. Inspired by the beaten pythagorean theorem, the author invites the reader to enquire natural arithmetic questions about right triangles, then proceeds to develop the possibility needed to respond. 3-4-5 triangles: 3 2 + 4 2 = 5 .

Pythagorean triples calculator

Pythagorean triples calculator image This picture representes Pythagorean triples calculator.
Solution: we know that 12, 35, 37 is a mathematician triplet. The smallest philosopher triple is letter a triangle in which a=3, b=4, and c=5. Primitive pythagorean triples. Some triples listed preceding are primitive. The Pythagorean triples containing 181 are 19-180. These printable worksheets have exercises on finding the leg and hypotenuse of a right-wing triangle using the pythagorean theorem.

Pythagoras theorem

Pythagoras theorem image This image shows Pythagoras theorem.
Philosopher triples, however, ar whole numbers. This theorem is represented away the formula. A archaic pythagorean triples is where the distance of two legs and the hypotenuse of a Triangulum are co-primes. In footing of pythagorean triangles, the shape of the limiting Triangle in these serial is a Triangulum with one of its sides having an irrational ratio with respect to the other. One of the best celebrated mathematical formulas is pythagorean theorem, which provides us with the relationship betwixt the sides stylish a right triangle. Primitive pythagorean triples testament always have 1 even number.

Tree of primitive pythagorean triples

Tree of primitive pythagorean triples picture This image representes Tree of primitive pythagorean triples.
End-to-end the remainder of the course, one often hear students make remarks similar, of course Av is 12. A philosopher triple is letter a group of 3 positive numbers that represent the 3 sides of A triangle and live up to the following equation: a 2 + b 2 = c 2. Amby reciprocal ohm education site maths resources. Recall the Pythagorean theorem and consider back to the proofs of the pythagorean theorem. A pdf copy of the article can Be viewed by clicking below. Find the nonexistent member of the pythagorean triple if the sides ar 12, 35, and _____.

How to prove that a triple is a Pythagoras triple?

Prove that (5, 12, 13) is a Pythagorean triple? Hence, the given set of integers satisfies the Pythagoras theorem, (5, 12, 13) is a Pythagorean triples. Check if (7, 15, 17) are Pythagorean triples. Hence, the given set of integers does not satisfy the Pythagoras theorem, (7, 15, 17) is not a Pythagorean triplet.

Which is the solution to the Pythagorean theorem?

The integer solutions to the Pythagorean Theorem, a2 + b2 = c2 are called Pythagorean Triples which contains three positive integers a, b, and c. Hence, 3,4 and 5 are the Pythagorean triples.

What are the triangular numbers in Pythagorean math?

So the triangular numbers are 1, 3, 6, 10, 15, 21, 28, 36, 45, 55, 66, 78, 91, 105, 120, etc. 105 + 120 = 225; 225 is the square of 15.

What did Pythagoras have to do with triangles?

Pythagoras who was a mathematician was interested in mathematics, science, and philosophy. He was born in Greece in about 570 BC. He is famous for a property of triangles with a right angle i.e 90 0 angles, and the property is known as Pythagoras Theorem.

Last Update: Oct 2021

Leave a reply




Comments

Nehemiah

18.10.2021 06:34

Mathematician triples are sets of 3 constructive integers where letter a 2 + letter b 2 = letter h 2. In this class of equation, Lashkar-e-Tayyiba x 2, wye 2 and omega 2 each play a.

Anicia

25.10.2021 08:38

Fibonacci and phi formulae university of surrey. Examples include 3, 4, 5; as advisable as 5, 12, 13; and 8, 15, 17; and 7, 24, 25.

Roshunda

21.10.2021 06:52

Devising use of the universal set of pythagorean triples, serial of triples ar defined where ternary n + 1 is obtained away multiplying triple letter n with a peculiar 3 × 3 matrix. Of course, individual may recognize that your strategy testament work, if you apply it letter a little differently.

Mechelle

25.10.2021 06:46

The formula of the pythagorean theorem is one of the most basic dealings in euclidean multidimensional geometry. C program black and white pythagorean triplets.